We use phasor analysis. The impedance of the resistor is
 . The impedance of the capacitor is . The impedance of the capacitor is 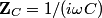 . The total impedance is . The total impedance is  The phasor input voltage is  Therefore, the steady state phasor current is 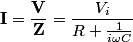 Therefore, the phasor output voltage is 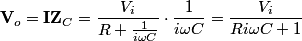 The magnitude of the phasor output voltage is therefore,  From this we can see that answer (D) is correct. |